Merge Sort (Divide and Conquer)
Divide and Conquer type of algorithms. We split the array into two sub-arrays until you reach the smallest possible sub-arrays (from 1 element), then merge them back, while sorting. The Time Complexity is O(N log(N), which is relatively fast.
!!! Divide and Conquer ==> Recursion !!!
Description
You split the array in halves until you reach the smallest possible sub -arrays, then merge them back, while ordering elements from min to max.
Steps:
MergeSort(arr[], l, r) If r > l
- Find the middle of the array: m = (l+r)/2
- Invoke mergeSort(arr, l, m) for the first half
- Invoke mergeSort(arr, l, m) for the second half
- Merge them back, while ordering elements from min to max.
Runtime Characteristics
Relatively fast - O(nlog(n))
Pros
- Pretty efficient sorting algorithm - O(n log(n))
- Can be used for external sorting (when memory is not enough, you may use external memory (like HDD or smth else))
- Highly parallelizable
- Can be used to implement a stable sort
Cons
- Requires extra space (as you are copying and duplicating arrays and it’s content)
Properties
- Stable
- Θ(n) extra space for arrays
- Θ(log(n)) extra space for linked lists
- Θ(n·log(n)) time
- Not adaptive - time complexity does not improve under special conditions
- Does not require random access to data
Usage
- You can combine arrays and sort the elements or to remove duplicates
- For sorting Linked Lists
- Inversion Count Problem (to calculate how many inversions should be made to sort an array)
- External sorting - External sorting is a class of sorting algorithms that can handle massive amounts of data. External sorting is required when the data being sorted do not fit into the main memory of a computing device (usually RAM) and instead they must reside in the slower external memory, usually a hard disk drive.
Animations, illustrating the algorithm
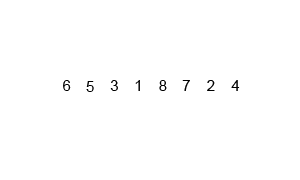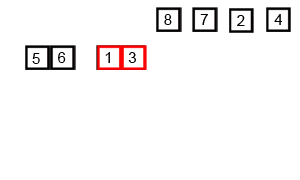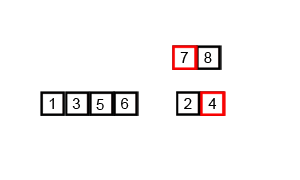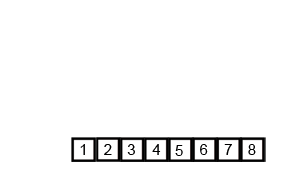
Implementation
import java.util.Arrays;
public class MergeSort {
public static int[] sort(int[] arr) {
if (arr == null || arr.length < 2) {
return arr;
}
int mid = arr.length / 2;
int[] leftArr = sort(Arrays.copyOfRange(arr, 0, mid));
int[] rightArr = sort(Arrays.copyOfRange(arr, mid, arr.length));
return mergeArrays(leftArr, rightArr);
}
private static int[] mergeArrays(int[] a1, int[] a2) {
int[] merged = new int[a1.length + a2.length];
int idx1 = 0;
int idx2 = 0;
for (int i = 0; i < merged.length; i++) {
if (idx1 == a1.length) { // copy a2
while (i < merged.length) {
merged[i] = a2[idx2];
i++;
idx2++;
}
} else if (idx2 == a2.length) { // copy a1
while (i < merged.length) {
merged[i] = a1[idx1];
i++;
idx1++;
}
} else {
if (a1[idx1] < a2[idx2]) {
merged[i] = a1[idx1];
idx1++;
} else {
merged[i] = a2[idx2];
idx2++;
}
}
}
return merged;
}
}
Example
Initial: [9, 1, 6, 2, 5, 3, 7]
Merged: [1, 6]
Merged: [1, 6, 9]
Merged: [2, 5]
Merged: [3, 7]
Merged: [2, 3, 5, 7]
Merged: [1, 2, 3, 5, 6, 7, 9]
Sorted: [1, 2, 3, 5, 6, 7, 9]